What Does A Circle Add Up To
In these lessons, nosotros review and summarise the properties of angles that tin be formed in a circumvolve and their theorems.
- Inscribed angles subtended by the aforementioned arc are equal.
- Primal angles subtended by arcs of the same length are equal.
- The key angle of a circle is twice whatever inscribed angle subtended past the same arc.
- Angle inscribed in semicircle is 90°.
- An bending between a tangent and a chord through the point of contact is equal to the angle in the alternate segment.
- The opposite angles of a cyclic quadrilateral are supplementary
- The exterior angle of a cyclic quadrilateral is equal to the interior opposite angle.
- A radius or diameter that is perpendicular to a chord divides the chord into two equal parts and vice versa.
- A tangent to a circumvolve is perpendicular to the radius drawn to the betoken of tangency.
- When two segments are drawn tangent to a circle from the aforementioned point exterior the circle, the segments are equal in length.
The following figures bear witness the Inscribed Angle Theorems and Angles in Circumvolve Theorems. Scroll downwards the folio for more examples and solutions of Inscribed Bending Theorems and Angles in Circle Theorems.
Inscribed Angles Subtended By The Same Arc Are Equal
The following diagram shows inscribed angles subtended past the same arc are equal.

∠x = ∠y because they are subtended by the aforementioned arc AEC.
Central Angles Subtended By Arcs Of The Same Length Are Equal
The post-obit diagram shows central angles subtended by arcs of the same length are equal.

∠ten = ∠y if arc AB = arc CD
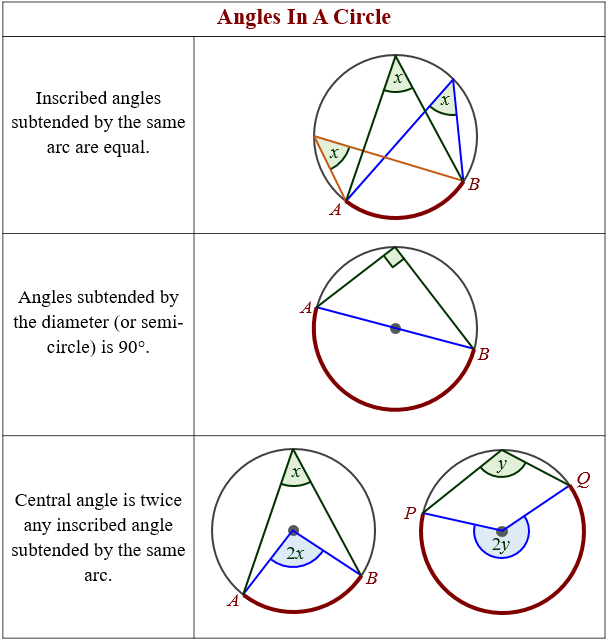
The Key Bending Is Twice The Inscribed Bending
The post-obit diagrams show the central angle of a circle is twice any inscribed angle subtended by the same arc.
Angle Inscribed In Semicircle Is 90°
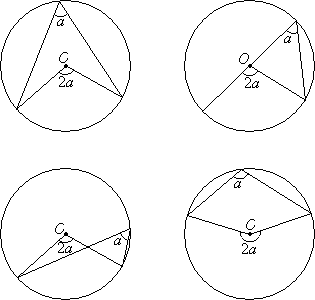
The following diagram shows the angle inscribed in semicircle is 90 degrees.
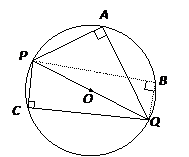
POQ is the diameter. ∠PAQ = ∠PBQ = ∠PCQ = ninety˚.
Alternate Segment Theorem
The diagram shows an angle between a tangent and a chord through the signal of contact is equal to the bending in the alternate segment.

The alternating segment theorem tells us that ∠CEA = ∠CDE
Angles In A Cyclic Quadrilateral
In a cyclic quadrilateral, the reverse angles are supplementary i.e. they add together up to 180°
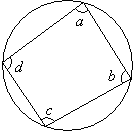
a + c = 180°, b + d = 180°
Exterior Angle Of A Circadian Quadrilateral Is Equal To The Interior Reverse Bending
The following diagram shows the exterior angle of a circadian quadrilateral is equal to the interior opposite angle.

The exterior angle ∠ADF is equal to the corresponding interior angle ∠ABC.
The exterior angle ∠DCE is equal to the corresponding interior angle ∠DAB.
Radius Perpendicular To A Chord Bisects The Chord
A radius or diameter that is perpendicular to a chord divides the chord into two equal parts and vice versa.
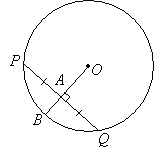
In the higher up circle, if the radius OB is perpendicular to the chord PQ then PA = AQ.
Tangent To A Circle Theorem
A tangent to a circle is perpendicular to the radius drawn to the point of tangency.

Two-Tangent Theorem
When 2 line segments are drawn tangent to a circle from the same point exterior the circle, the segments are equal in length.
In the post-obit diagram:
If AB and AC are two tangents to a circumvolve centred at O, then:
- the tangents to the circle from the external point A are equal.
- OA bisects the ∠BAC betwixt the two tangents.
- OA bisects the ∠BOC between the two radii to the points of contact.
- triangle AOB and triangle AOC are congruent right triangles.
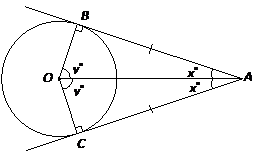
Videos
This video gives a review of the post-obit circumvolve theorems: arrow theorem, bow theorem, cyclic quadrilateral, semi-circle, radius-tangent theorem, alternating segment theorem, chord centre theorem, dual tangent theorem.
- Show Video Lesson
This video gives a review of the post-obit circle theorems: aforementioned segment, subtended by arc, angle in semicircle, tangents equal length, radius tangent, alternate segment, bifurcate chord, cyclic quadrilateral. It too includes the proofs of the theorem.
- Bear witness Video Lesson
Try the free Mathway calculator and trouble solver beneath to practice various math topics. Try the given examples, or type in your own problem and cheque your answer with the step-past-step explanations.

We welcome your feedback, comments and questions nigh this site or page. Delight submit your feedback or enquiries via our Feedback page.
What Does A Circle Add Up To,
Source: https://www.onlinemathlearning.com/angles-circle.html
Posted by: cobbnoversetied.blogspot.com


0 Response to "What Does A Circle Add Up To"
Post a Comment